Alright folks, let’s dive straight into the world of geometry because today we’re talking about something pretty cool—heptagon sides. You’ve probably heard about triangles, squares, and hexagons, but have you ever wondered about the mysterious seven-sided figure? A heptagon is not just another polygon; it’s a shape with some seriously interesting properties. So buckle up, because we’re about to break it down for you in a way that’s easy to digest and super fun!
Now, if you’re anything like me, you might be thinking, “Why should I care about heptagon sides?” Well, my friend, understanding polygons like heptagons isn’t just for math nerds. It’s about appreciating the beauty of shapes in our everyday lives, from architecture to nature. So whether you’re a student, a teacher, or just someone curious about geometry, this article’s got you covered.
Before we jump into the nitty-gritty, let’s set the stage. We’ll explore everything from the basic definition of a heptagon to its real-world applications, with a sprinkle of fun facts along the way. By the end of this, you’ll be a heptagon expert, ready to impress your friends and family with your newfound knowledge. Let’s get started!
Read also:Mastering Remoteiot Web Ssh A Raspberry Pi Guide To Download And Use Freely
What Exactly is a Heptagon?
Let’s start with the basics. A heptagon is a polygon with seven sides and seven angles. The word “heptagon” comes from the Greek words “hepta,” meaning seven, and “gonia,” meaning angle. So, essentially, it’s a shape with seven straight edges that meet at seven vertices. Now, don’t let the fancy terminology scare you—this is geometry at its simplest.
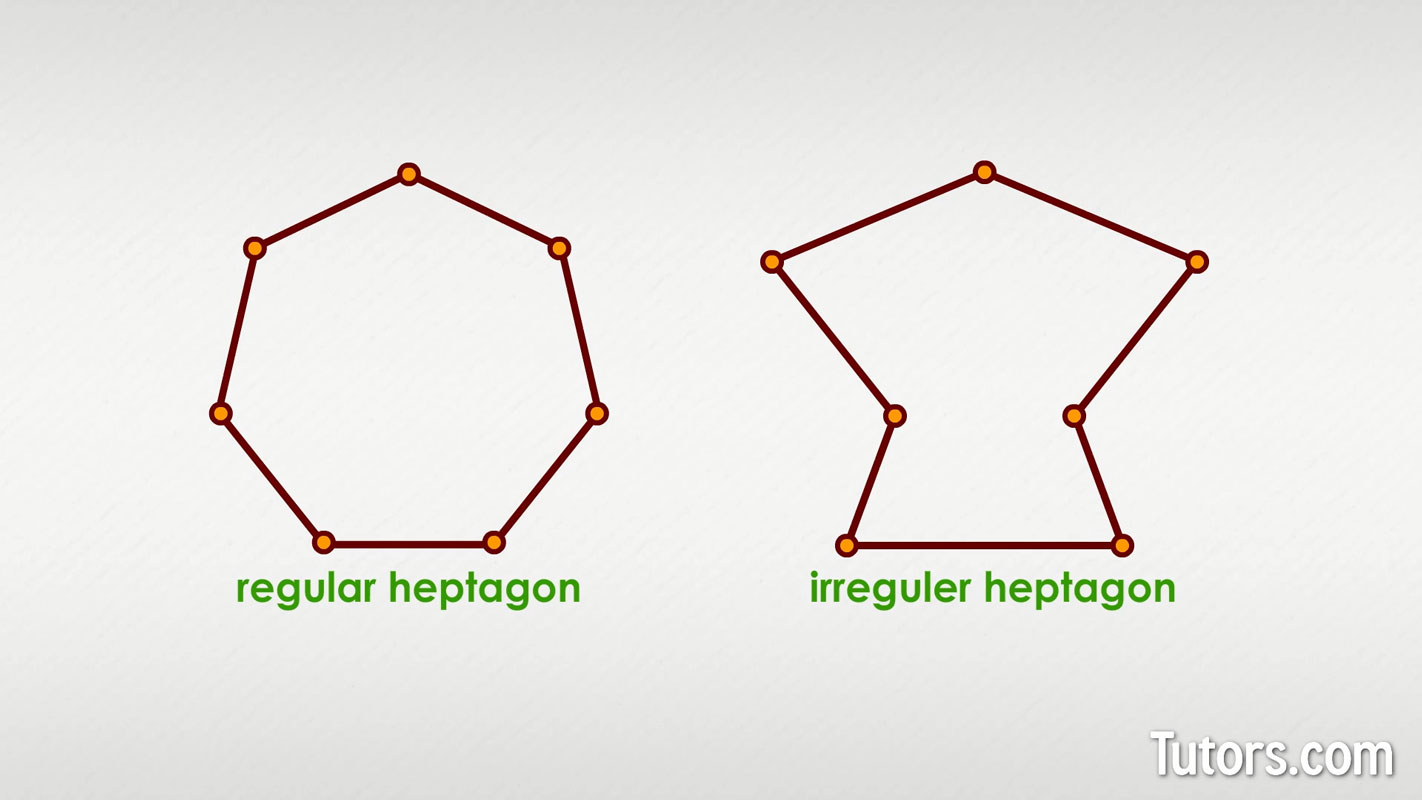
There are two main types of heptagons: regular and irregular. A regular heptagon has all sides and angles equal, while an irregular heptagon doesn’t follow these rules. Think of it like this: a regular heptagon is like a perfectly symmetrical flower, while an irregular heptagon is more like a funky, asymmetrical art piece.
How Many Sides Does a Heptagon Have?
Okay, here’s where things get interesting. A heptagon, by definition, has exactly seven sides. That’s what makes it unique among polygons. But why stop at just the number of sides? Let’s dig deeper into the properties of these sides and what makes them special.
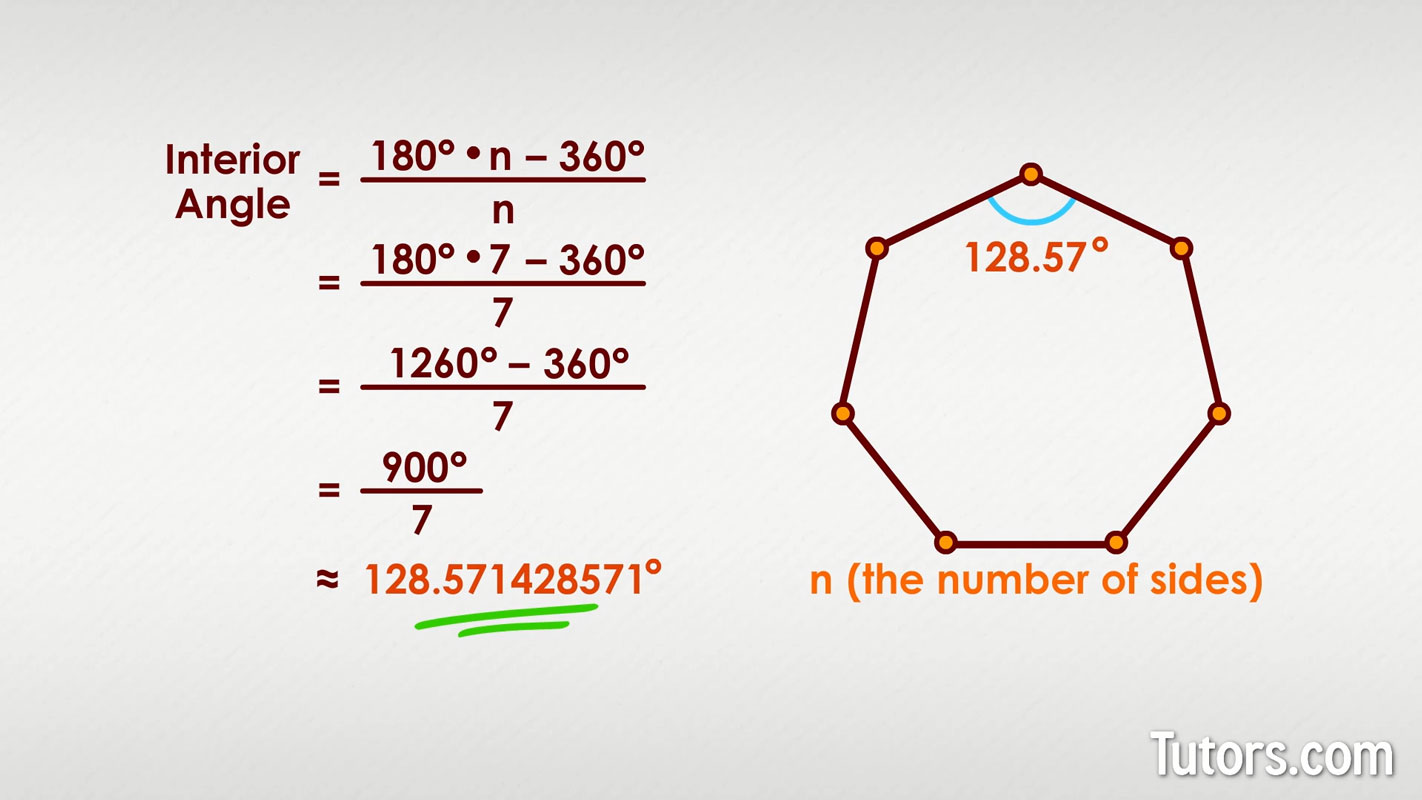
Each side of a regular heptagon is equal in length, and each interior angle measures approximately 128.57 degrees. This symmetry gives the heptagon its distinctive shape and makes it a favorite in geometry textbooks and real-world designs.
Why Are Heptagon Sides Important?
Heptagon sides aren’t just random lines; they hold mathematical significance. For starters, the length of each side determines the overall size and shape of the heptagon. In practical terms, this means that knowing the length of one side allows you to calculate the perimeter, area, and other properties of the heptagon.
Additionally, heptagon sides play a crucial role in various fields, from architecture to computer graphics. Architects use heptagons to create unique building designs, while graphic designers leverage their symmetry to craft visually appealing patterns.
Read also:Hdhub4ucom 2024 Your Ultimate Destination For Highquality Entertainment
Calculating the Perimeter of a Heptagon
Now, let’s talk numbers. If you want to calculate the perimeter of a heptagon, all you need is the length of one side. Since a regular heptagon has seven equal sides, the formula is simple: Perimeter = 7 × Side Length. Easy peasy, right?
Here’s an example: If the length of one side is 5 cm, the perimeter would be 7 × 5 = 35 cm. See? Math doesn’t have to be complicated!
Breaking Down the Formula
Let’s break this down further. The perimeter of a polygon is essentially the sum of all its sides. In the case of a heptagon, since all sides are equal, you can simply multiply the length of one side by the number of sides, which is seven. This formula works for both regular and irregular heptagons, though the latter requires a bit more calculation.
For irregular heptagons, you’ll need to add up the lengths of all seven sides individually. It’s like putting together a puzzle—one piece at a time.
Exploring the Area of a Heptagon
While the perimeter is all about the outside, the area focuses on the inside. Calculating the area of a heptagon requires a bit more effort, but it’s definitely doable. For a regular heptagon, the formula is: Area = (7 × Side²) / (4 × tan(π/7)).
Don’t worry if that looks intimidating. Let’s simplify it. Imagine you have a heptagon with a side length of 10 cm. Plugging that into the formula gives you: Area = (7 × 10²) / (4 × tan(π/7)) ≈ 172.05 square centimeters. Not too shabby!
Real-World Applications of Heptagon Area
Understanding the area of a heptagon isn’t just theoretical; it has practical applications. For instance, architects use this knowledge to design buildings with heptagonal floors or ceilings. Similarly, engineers might calculate the area of a heptagonal component in machinery to ensure it fits perfectly.
Even in nature, you’ll find examples of heptagonal shapes. Some flowers and crystals exhibit heptagonal symmetry, making them a fascinating subject for scientists and mathematicians alike.
Types of Heptagons
Not all heptagons are created equal. As mentioned earlier, there are two main types: regular and irregular. But did you know there are also convex and concave heptagons? Let’s take a closer look.
- Regular Heptagon: All sides and angles are equal.
- Irregular Heptagon: Sides and angles vary in length and measure.
- Convex Heptagon: All interior angles are less than 180 degrees.
- Concave Heptagon: At least one interior angle is greater than 180 degrees.
Each type has its own unique properties and applications, making heptagons a versatile shape in both mathematics and design.
How to Identify a Heptagon
So, how can you tell if a shape is a heptagon? Look for these key characteristics:
- It has exactly seven sides.
- It has seven vertices (corners).
- It can be regular or irregular, convex or concave.
Remember, a heptagon doesn’t have to be perfect to qualify as one. Even if the sides and angles aren’t equal, as long as there are seven of them, it’s still a heptagon.
Heptagons in Nature and Beyond
Heptagons aren’t just confined to geometry textbooks; they appear in nature and human creations alike. From the petals of certain flowers to the structure of some crystals, heptagonal shapes are all around us.
One fascinating example is the arrangement of atoms in certain molecules. Some compounds form heptagonal rings, giving them unique chemical properties. In architecture, heptagons are used to create visually striking buildings and monuments.
Fun Facts About Heptagons
Here are a few fun facts to impress your friends:
- The heptagon is one of the few polygons that cannot be constructed using only a compass and straightedge.
- Heptagons have been used in art and design for centuries, symbolizing balance and harmony.
- In some cultures, the number seven is considered sacred, making heptagons a powerful symbol.
Who knew a simple seven-sided shape could be so intriguing?
Common Misconceptions About Heptagon Sides
Let’s clear up some common misconceptions about heptagon sides. One of the biggest myths is that all heptagons are regular. While regular heptagons are the most well-known, irregular heptagons are just as valid and interesting.
Another misconception is that heptagons are rare in real life. In reality, they appear in a variety of contexts, from nature to architecture. So the next time you see a seven-sided shape, don’t dismiss it as unusual—embrace its uniqueness!
How to Avoid Mistakes When Working with Heptagons
When working with heptagons, it’s easy to make mistakes, especially if you’re not familiar with their properties. Here are a few tips to help you avoid common pitfalls:
- Double-check your measurements before calculating perimeter or area.
- Be mindful of whether the heptagon is regular or irregular, as this affects the formulas you use.
- Use diagrams and visual aids to better understand the shape and its properties.
By following these tips, you’ll be well on your way to mastering heptagons.
Conclusion: Why Heptagon Sides Matter
And there you have it, folks—a comprehensive guide to heptagon sides. From their definition and properties to their real-world applications, we’ve covered it all. Heptagons may not be as famous as triangles or squares, but they’re just as fascinating and important in their own right.
So the next time you encounter a seven-sided shape, take a moment to appreciate its beauty and complexity. And don’t forget to share this article with your friends and family. Who knows? You might just inspire someone to explore the world of geometry further.
Until next time, keep learning, keep exploring, and keep loving math!
Table of Contents
- What Exactly is a Heptagon?
- How Many Sides Does a Heptagon Have?
- Why Are Heptagon Sides Important?
- Calculating the Perimeter of a Heptagon
- Exploring the Area of a Heptagon
- Types of Heptagons
- Heptagons in Nature and Beyond
- Common Misconceptions About Heptagon Sides
- Fun Facts About Heptagons
- Conclusion: Why Heptagon Sides Matter